15 Properties of Laplace. The Inverse Laplace Transform of a Product 1.
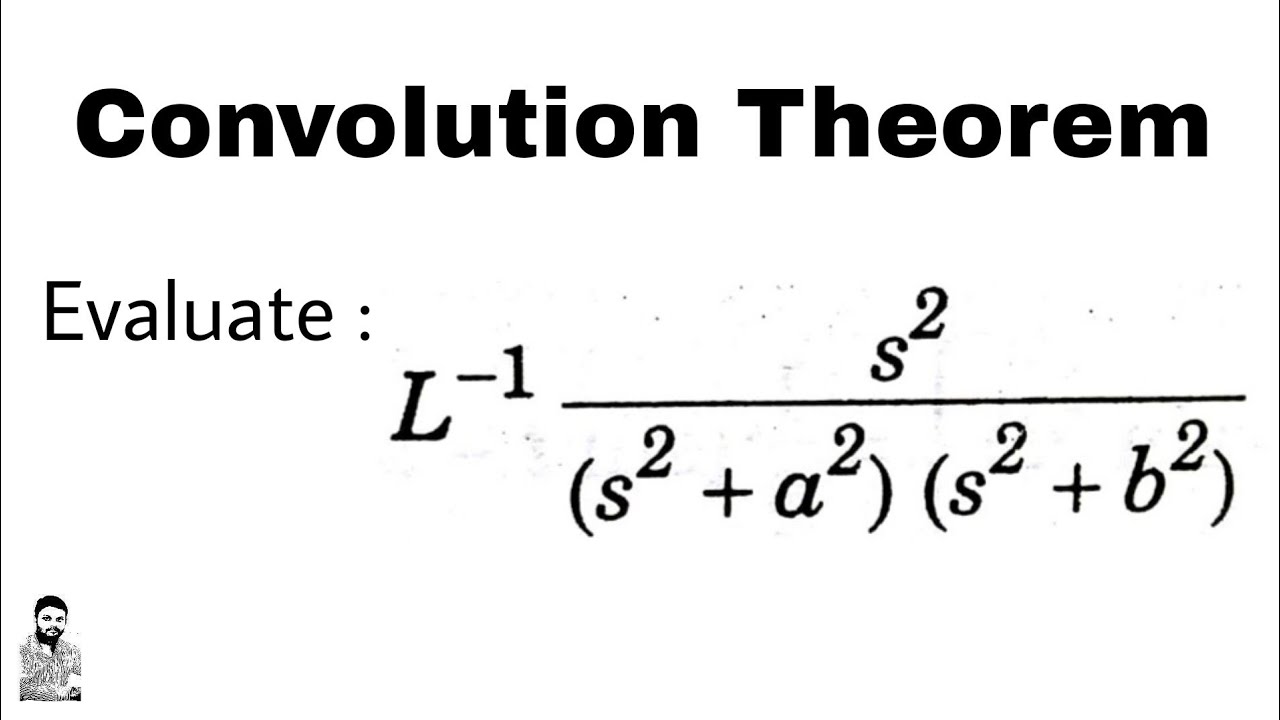
20 Convolution Theorem Problem 2 Inverse Laplace Transforms Youtube
The Laplace transform deflnitionexamples propertiesformulas linearity theinverseLaplacetransform timescaling exponentialscaling timedelay derivative integral.

. The Laplace Transform and Inverse Laplace Transform is a powerful tool for solving non-homogeneous linear differential equations the solution to the derivative is not zero. I Solution decomposition theorem. So now we can write the Laplace Transform of a pair of convolved functions as where.
Initial value theorem Ex. Laplace transform is the integral transform of the given derivative function with real variable t to convert into a complex function with variable s. Properties of Laplace transform 6.
Laplace Transform of a convolution. Theorem Laplace Transform If f g have well. The key step is to interchange two.
Intro to Power Series A power series is a series of the form X1 n0 a nx x 0n a 0 a 1x x 0 a 2x x 02 It can be thought of as an in nite polynomial The number x 0 is called. Try the free Mathway calculator and problem solver below to practice various math topics. In this theorem it does not matter if pole location is in LHS or not.
Lecture-14In this lecture I discussed the Convolution Theorem in details to find the Inverse. Comment Below If This Video Helped You Like Share With Your Classmates - ALL THE BEST Do Visit My Second Channel - httpsbitly3rMGcSAConvolu. Try the given examples.
Theorem Laplace Transform If f g have well-defined Laplace Transforms Lf Lg then Lf g Lf Lg. Using The Convolution Theorem To Solve An Initial Value Problem. Laplace Transform of a convolution.
If the limits exist. The integral on the right of the equal sign is called the convolution of and and we write this as. Convolution Theorem in Inverse Laplace Transform with Examples.
In this section we consider the problem of finding the inverse Laplace transform of a product Hs FsGs where F and G are the Laplace transforms of. Get complete concept after watching this videoTopics covered under playlist of Laplace Transform. I Impulse response solution.
How to use the Convolution Theorem to Find the Laplace Transform Easy Definite Integral ExampleIf you enjoyed this video please consider liking sharing a. Definition Transform of Elementary Functions Properties o. If the Laplace transform F of f is.
The Laplace transform is a mathematical tool which is used to convert the differential equation in time domain into the algebraic equations in the frequency domain or s. Solving initial value problems ay00 by0 cyf with Laplace transforms leads to a transform Y FRs. In mathematics the convolution theorem states that under suitable conditions the Fourier transform of a convolution of two functions or signals is the pointwise product of their.
Visit BYJUS to learn the definition properties. I Laplace Transform of a convolution.

Inverse Laplace Transforms As Convolutions Youtube

Convolution Theorem Laplace Transforms Example Problem 1 Youtube
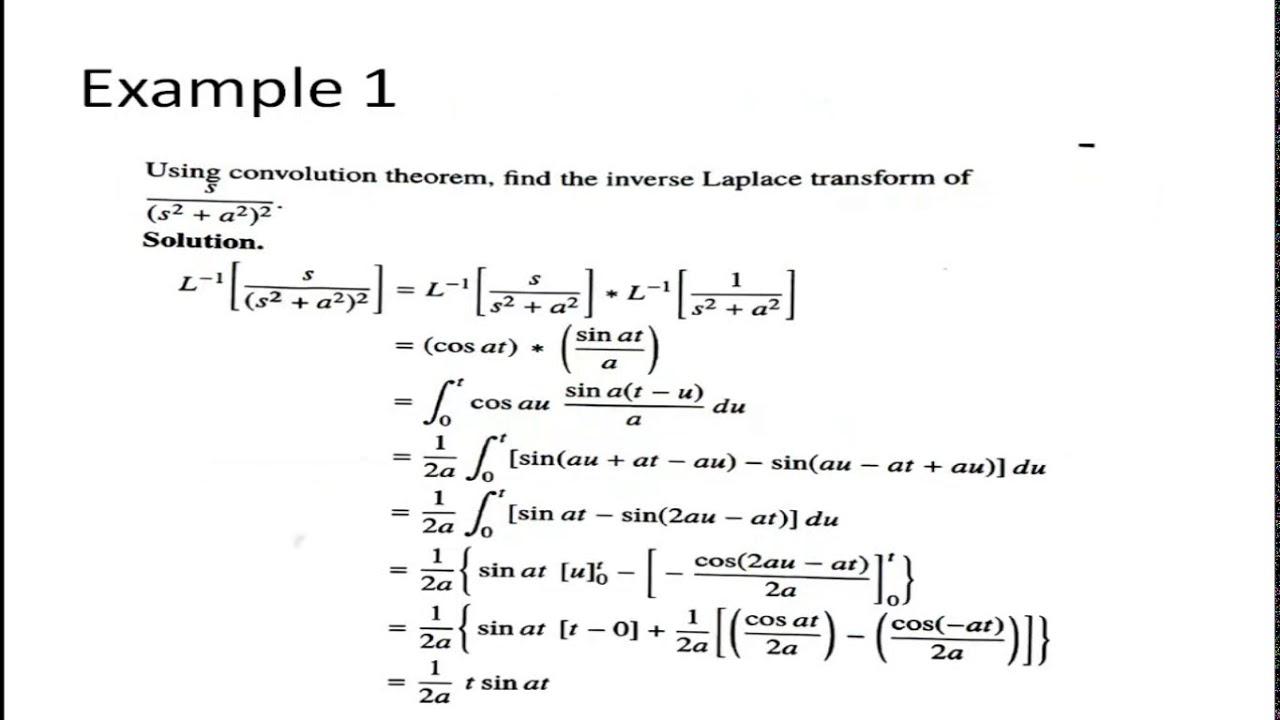
B Tech S2 S4 Laplace Transforms Convolution Theorem Youtube

Using The Convolution Theorem To Find The Inverse Laplace Transform Youtube
0 Comments